how to draw hyperbola by rectangular method

Find the centre of the hyperbola Locate the vertices and construct the corresponding vertical lines Determine the co-vertices and construct the corresponding horizontal lines This creates a rectangle Draw two diagonal lines for this rectangle. These are the asymptotes of the hyperbola Sketch the curves

how to find the equation of a hyperbola

Decide whether the transverse axis is parallel to the x-axis or y-axis Identify the centre of the hyperbola, (h, k) using the Midpoint Formula given coordinates of the vertices Evaluate a 2 by solving the length of the transverse axis, 2a . This is given by the distance between the two vertices Evaluate c 2 by using the coordinates of the given foci and the values of h and k determined in Step 2 Solve for b 2 Substitute a 2 , b 2 , h and k into the standard form established in Step 1

Hyperbolas

TABLE OF CONTENTS :

TABLE OF CONTENTS

Lerne mit deinen Freunden und bleibe auf dem richtigen Kurs mit deinen persönlichen Lernstatistiken

Jetzt kostenlos anmelden

Nie wieder prokastinieren mit unseren Lernerinnerungen.

Jetzt kostenlos anmelden

A hyperbola is a type of conic section made up of two curves that resemble parabolas (although they are not). These pairs of curves, also called branches, can either open up and down or left and right. Additionally, each curve contains a vertex.

In this article, we aim to look at the properties of hyperbolas and identity equations that describe such conic sections. By familiarizing ourselves with these concepts, we can ultimately graph hyperbolas given a pair of coordinates.

A hyperbola is the set H of all points P in a plane where the absolute value of the difference in distance between two fixed points, called the foci, F₁ and F₂, is constant, k, that is

$H = {P : | | P F_{1} | - | P F_{2} | | = k, k \in ℝ}$ .

We shall further look into the components of this graph in the following section.

To visualize this, observe the graph below.

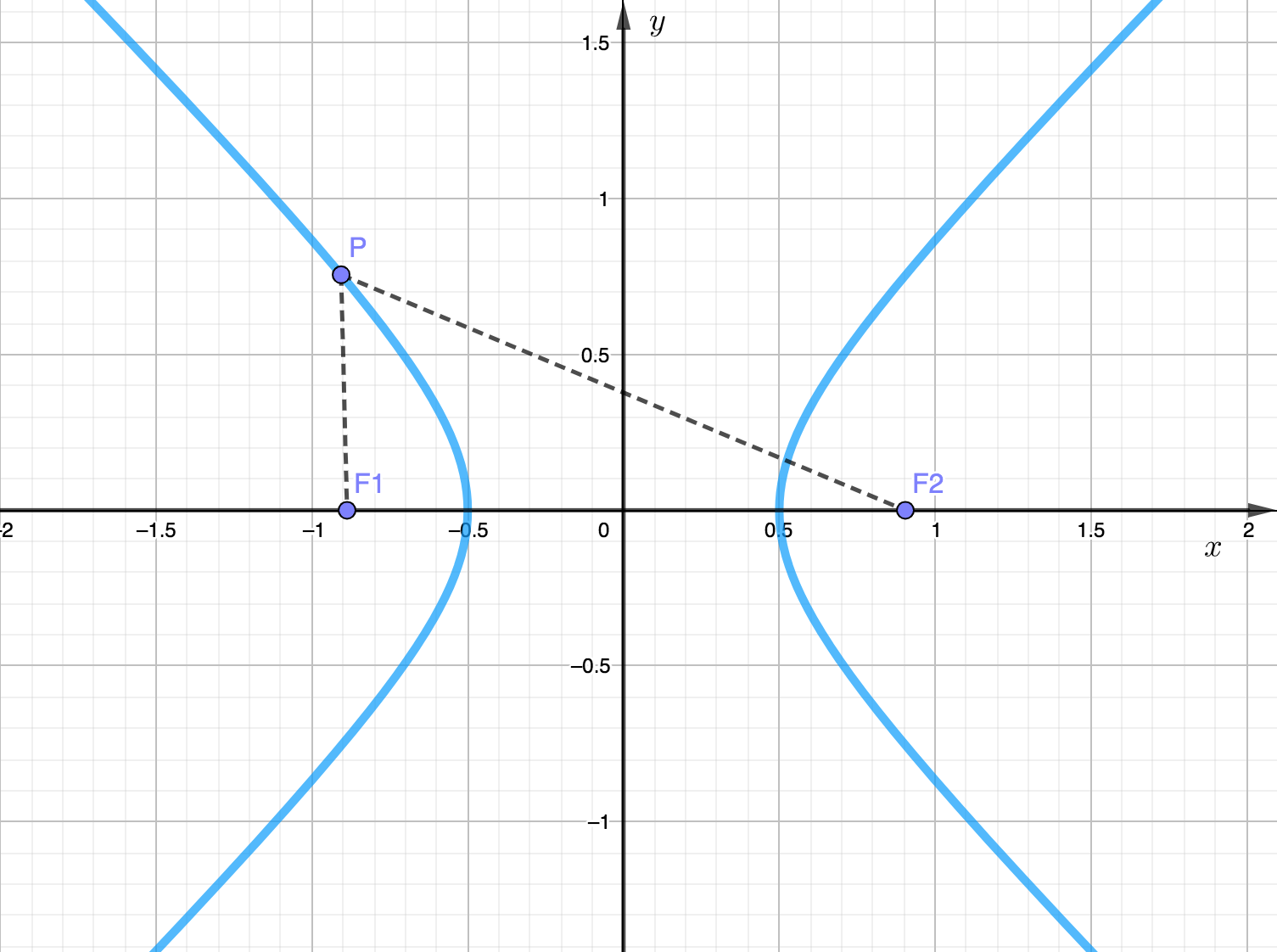
Notice that unlike other types of conic sections, hyperbolas are made up of two branches rather than one.

Geometrical Components of a Hyperbola

From a geometric point of view, a hyperbola is produced when a plane cuts parallel to the cone axis of a double-napped cone.

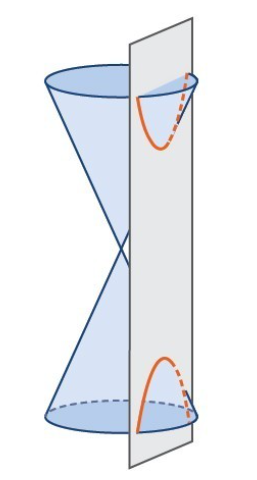
Let's explore more closely a graphical representation of a hyperbola. Here, we introduce more vital components that make up a hyperbola.

Every hyperbola has two axes of symmetry: the transverse axis and the conjugate axis
The conjugate axis (the y-axis) is a line perpendicular to the transverse axis and contain the co-vertices.
The transverse axis (the x-axis) is a line that passes through the centre of the hyperbola. The foci (focus F₁ and focus F₂₎ lie on the transverse line. The vertices are the points of intersection of both branches of the hyperbola with the transverse line. Both foci and vertices are symmetrical in relation to the conjugate axis, which implies that they'll have the same x-coordinate but with opposite signs.
The centre is the midpoint of the transverse axis and conjugate axis. This is where the two lines intersect.
Every hyperbola has two asymptotes (red dashed lines) that pass through the centre. As a hyperbola moves away from its centre, the branches approach these asymptotes. By definition, the branches of the hyperbola will never intersect it's asymptotes.
The central rectangle (orange dashed lines) is centred at the origin. The sides pass through each vertex and co-vertex.

It is helpful to identify this when graphing the hyperbola and its asymptotes. To draw the asymptotes of the hyperbola, all we have to do is extend the diagonals of the central rectangle.

Equations of Hyperbolas

Let us now derive the equation of a hyperbola centred at the origin.

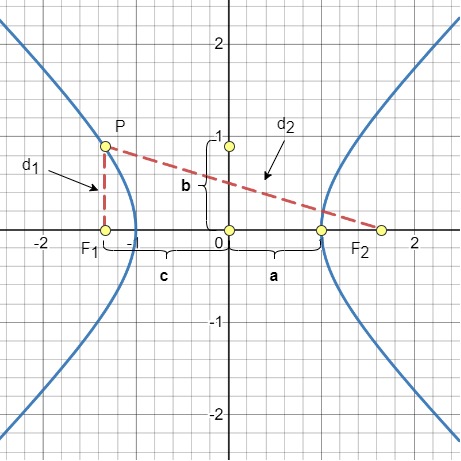
Let P = (x, y), and the foci of a hyperbola centred at the origin be F₁ = (–c, 0) and F₂ = (c, 0)

From the plot above, is a vertex of the hyperbola, and so the distance from to $(a, 0)$ is $a - (- c) = a + c$ . Similarly, the distance from to is $c - a$ .

The sum of the distances from the foci to the vertex is $(a + c) - (c - a) = 2 a.$ Let P(x, y) be a point on the hyperbola we want to investigate. From here, we can define d₁ and d₂ by:

d₁ = distance from (c, 0) to (x, y)

d₂ = distance from (–c, 0) to (x, y)

$By definition, a hyperbola is the set of all points where the difference of the distance between (x, y) to the foci is constant. Thus, d2 - d1 is constant for any point (x, y) on the hyperbola. We know that the difference of these distances is 2a for the vertex (a, 0). So, d2 - d1 = 2a.$

$To find the equation of a hyperbola, we shall make use of the Distance Formula and solve our expression algebraically.$

$The Distance Formula$

$Recall that the Distance Formula for two points (x1, y1) and (x2, y2) is given by$

$d = \sqrt{{(x_{2} - x_{1})}^{2} + (y}$

$By the Distance Formula between (-c, 0) and (c, 0)$

$d_{2} - d_{1} = \sqrt{(x - {(- c))}^{2} + {(y - 0)}^{2}} - \sqrt{{(x - c)}^{2} + {(y - 0)}^{2}} = 2 a \Rightarrow \sqrt{(x + {c)}^{2} + y^{2}} - \sqrt{{(x - c)}^{2} + y^{2}} = 2 a \Rightarrow \sqrt{(x + {c)}^{2} + y^{2}} = 2 a + \sqrt{{(x - c)}^{2} + y^{2}}$

Squaring both sides, we obtain

$\Rightarrow (x + {c)}^{2} + y^{2} = {(2 a + \sqrt{{(x - c)}^{2} + y^{2}})}^{2} \Rightarrow x^{2} + 2 c x + c^{2} + y^{2} = 4 a^{2} + 4 a \sqrt{{(x - c)}^{2} + y^{2}} + {(x - c)}^{2} + y^{2}$

Expanding the binomials and cancelling like terms,

$\Rightarrow x^{2} + 2 c x + c^{2} + y^{2} = 4 a^{2} + 4 a \sqrt{{(x - c)}^{2} + y^{2}} + x^{2} - 2 c x + c^{2} + y^{2} \Rightarrow 4 c x - 4 a^{2} = 4 a \sqrt{{(x - c)}^{2} + y^{2}}$

Now dividing both sides by 4 and squaring both sides,

$\Rightarrow c x - a^{2} = a \sqrt{{(x - c)}^{2} + y^{2}} \Rightarrow {(c x - a^{2})}^{2} = {(a \sqrt{{(x - c)}^{2} + y^{2}})}^{2}$

Expanding this and cancelling like terms again yields,

$\Rightarrow c^{2} x^{2} - 2 a^{2} c x + a^{4} = a^{2} (x^{2} - 2 c x + c^{2} + y^{2}) \Rightarrow c^{2} x^{2} - 2 a^{2} c x + a^{4} = a^{2} x^{2} - 2 a^{2} c x + a^{2} c^{2} + a^{2} y^{2} \Rightarrow c^{2} x^{2} - a^{2} x^{2} - a^{2} y^{2} = a^{2} c^{2} - a^{4}$

This simplifies to

$\Rightarrow x^{2} (c^{2} - a^{2}) - a^{2} y^{2} = a^{2} (c^{2} - a^{2})$

$The distance between the foci is 2c with c2 = a2 + b2. Thus, we can set b2 = c2 - a2. In doing so, we obtain$

$\Rightarrow x^{2} b^{2} - a^{2} y^{2} = a^{2} b^{2}$

Now dividing both sides by a²b², the equation for the hyperbola becomes

$\Rightarrow \frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1$

as required! Below is a worked example that demonstrates the use of the Distance Formula in regards to hyperbolas.

Determine the equation of the hyperbola represented by the graph below.

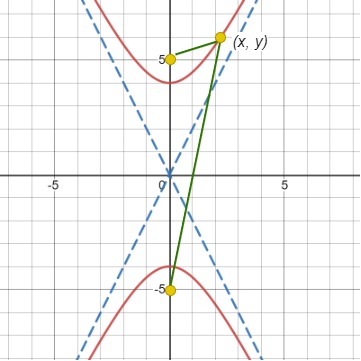
Solution

The hyperbola below has foci at (0 , –5) and (0, 5) while the vertices are located at (0, –4) and (0, 4). The distance between these two coordinates is 8 units.

Thus, the difference between the distance from any point (x, y) on the hyperbola to the foci is 8 or –8 units, depending on the order in which you subtract.

Using the Distance Formula, we obtain the equation of the hyperbola as follows.

Let,

d₁ = distance from (0, 5) to (x, y)

d₂ = distance from (0, –5) to (x, y)

$d_{2} - d_{1} = \pm 8 \Rightarrow \sqrt{{(y - 5)}^{2} + x^{2}} - \sqrt{{(y + 5)}^{2} + x^{2}} = \pm 8 \Rightarrow \sqrt{{(y - 5)}^{2} + x^{2}} = \pm 8 + \sqrt{{(y + 5)}^{2} + x^{2}} \Rightarrow {(y - 5)}^{2} + x^{2} = {(\pm 8 + \sqrt{{(y + 5)}^{2} + x^{2}})}^{2} \Rightarrow y^{2} - 10 y + 25 + x^{2} = 64 + 16 \sqrt{{(y + 5)}^{2} + x^{2}} + {(y + 5)}^{2} + x^{2} \Rightarrow y^{2} - 10 y + 25 + x^{2} = 64 \pm 16 \sqrt{{(y + 5)}^{2} + x^{2}} + y^{2} + 10 y + 25 + x^{2} \Rightarrow - 20 y - 64 = \pm 16 \sqrt{{(y + 5)}^{2} + x^{2}} \Rightarrow 5 y + 16 = \pm 4 \sqrt{{(y + 5)}^{2} + x^{2}} \Rightarrow {(5 y + 16)}^{2} = 16 {(\sqrt{{(y + 5)}^{2} + x^{2}})}^{2} \Rightarrow 25 y^{2} + 160 y + 256 = 16 (y^{2} + 10 y + 25 + x^{2}) \Rightarrow 25 y^{2} + 160 y + 256 = 16 y^{2} + 160 y + 400 + 16 x^{2} \Rightarrow 9 y^{2} - 16 x^{2} = 144$

By dividing the expression by $9 \times 16,$ our final yield becomes

$\Rightarrow \frac{y^{2}}{16} + \frac{x^{2}}{9} = 1$

Properties of Hyperbolas

Let us now move on to the properties of hyperbolas. There are two cases to consider here:

Case 1: Hyperbolas Centred at the Origin (0, 0)

Property	Centre (0,0)
Standard Form of Equation	$\frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1$	$\frac{y^{2}}{a^{2}} - \frac{x^{2}}{b^{2}} = 1$
General Plot
Opening	Opens left and right	Opens up and down
Direction of Transverse Axis	Horizontal	Vertical
Foci	(c, 0), (-c, 0)	(0, c), (0, -c)
Vertices	(a, 0), (-a, 0)	(0, a), (0, -a)
Length of Transverse Axis	2a units	2a units
Length of Conjugate Axis	2b unites	2b units
Equation of Asymptotes	$y = \pm \frac{b}{a} x$	$y = \pm \frac{a}{b} x$

Case 2: Hyperbolas Centred at (h, k)

Property	Centre (h,k)
Standard Form of Equation	$\frac{{(x - h)}^{2}}{a^{2}} - \frac{{(y - k)}^{2}}{b^{2}} = 1$	$\frac{{(y - k)}^{2}}{a^{2}} - \frac{{(x - h)}^{2}}{b^{2}} = 1$
General Plot
Vertices	(h + a, k), (h - a, k)	(h, k + a), (h, k - a)
Foci	(h + c, k), (h - c, k)	(h, k + c), (h, k - c)
Equation of Asymptotes	$y = k \pm \frac{b}{a} (x - h)$	$y = k \pm \frac{a}{b} (x - h)$

Locating the Foci and Vertices of a Given Hyperbola

Identify the foci and vertices for the hyperbola $\frac{y^{2}}{49} - \frac{x^{2}}{32} = 1$ .

Solution

The equation is of the form

$\frac{y^{2}}{a^{2}} - \frac{x^{2}}{b^{2}} = 1$

Thus, the transverse axis lies on the y-axis. The centre is at the origin, so the y-intercepts are the vertices of the graph. We can thus find the vertices by setting x = 0 and solve for y as below.

$\frac{y^{2}}{49} - \frac{{(0)}^{2}}{32} = 1 \Rightarrow \frac{y^{2}}{49} = 1 \Rightarrow y^{2} = 49 \Rightarrow y = \pm \sqrt{49} = \pm 7$

The foci are located at $(0, \pm c)$ and by the relationship between a, b and c established before, we obtain

$c^{2} = a^{2} + b^{2} \Rightarrow c = \pm \sqrt{a^{2} + b^{2}} = \pm \sqrt{49 + 32} = \pm \sqrt{81} = \pm 9$

Thus, the vertices are (0, –7) and (0, 7) while the foci are (0, –9) and (0, 9). The graph is shown below.

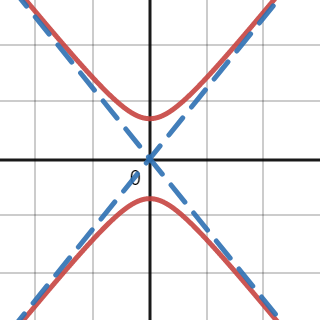
Identify the foci and vertices for the hyperbola $\frac{{(y - 2)}^{2}}{9} - \frac{{(x - 3)}^{2}}{25} = 1$ .

Solution

The equation is of the form

$\frac{{(y - k)}^{2}}{a^{2}} - \frac{{(x - h)}^{2}}{b^{2}} = 1$

Thus, the transverse axis lies on the y-axis. Here, h = 3 and k = 2, so the centre is at (3, 2). To find the vertices, we shall make use of the formula below.

$(h, k \pm a) \Rightarrow (3, 2 \pm \sqrt{9}) = (3, 2 \pm 3)$

The foci are located at $(0, \pm c)$ and using $c^{2} = a^{2} + b^{2}$ as before, we obtain

$c = \pm \sqrt{9 + 25} = \pm \sqrt{34} \approx \pm 5.83 (c o r r e c t t o t w o d e c i m a l p l a c e s)$

Thus, the vertices are (3, –1) and (3, 5) while the foci are (0, –5.83) and (0, 5.83). The graph is shown below.

The equation for the asymptotes can be found using the formula given in the table. Give it a try for these examples! It is always helpful to sketch the asymptotes first before drawing the two branches of a hyperbola.

Finding the Equation of a Hyperbola Given the Foci and Vertices

Express the following hyperbola in standard form given the following foci and vertices.

$V e r t i c e s : (\pm 6, 0) F o c i : (\pm 2 \sqrt{10}, 0)$

Solution

Notice that the vertices and foci are on the x-axis. Hence, the equation of the hyperbola will take the form

$\frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1$

Since the vertices are $(\pm 6, 0),$ then

$a = 6 \Rightarrow a^{2} = 36$

Given the foci are $(\pm 2 \sqrt{10}, 0),$ then

c = 2 \sqrt{10} \Rightarrow c^{2} = 40

Solving for b² we obtain

$b^{2} = c^{2} - a^{2} = 40 - 36 \Rightarrow b^{2} = 4$

Now that we have found a² and b², we can substitute this into the standard form as

$\frac{x^{2}}{36} - \frac{y^{2}}{4} = 1$

The graph is shown below.

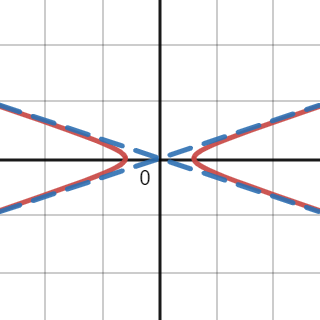
Express the following hyperbola in standard form given the following foci and vertices.

$V e r t i c e s : (1, - 2) a n d (1, 8) F o c i : (1, - 10) a n d (1, 16)$

Solution

The vertices and foci have the same x-coordinates, so the transverse axis is parallel to the y-axis. The equation of the hyperbola will thus take the form

$\frac{{(y - k)}^{2}}{a^{2}} - \frac{{(x - h)}^{2}}{b^{2}} = 1$

We must first identify the centre using the midpoint formula. The centre lies between the vertices (1, –2) and (1, 8), so

$(h, k) = (\frac{1 + 1}{2}, \frac{- 2 + 8}{2}) = (1, 3)$

The length of the transverse axis, 2a, is bounded by the vertices. To find a² we must evaluate the distance between the y-coordinates of the vertices.

$2 a = |- 2 - 8| = |- 10| = 10 \Rightarrow a = 5 \Rightarrow a^{2} = 25$

The coordinates of the foci are

$(h, k \pm c) \Rightarrow (h, k - c) = (1, - 10) a n d (h, k + c) = (1, 16)$

Using k + 3 = 16 and substituting k = 3, we obtain

$c = 13 \Rightarrow c^{2} = 169$

Thus, we can solve for b² by

$b^{2} = c^{2} - a^{2} \Rightarrow b^{2} = 169 - 25 = 144$

Finally, substituting these values into the standard form, we obtain

$\frac{{(y - 3)}^{2}}{25} - \frac{{(x - 1)}^{2}}{144} = 1$

The graph is shown below.

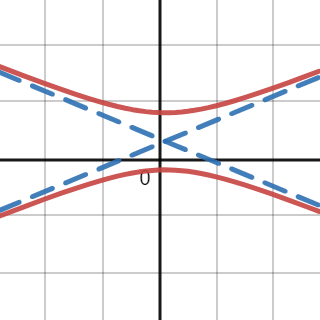
Graphing Hyperbolas

In this final section, we shall graph hyperbolas using the concepts introduced throughout this lesson.

Graph an Equation in Standard Form

Let us return to our previous examples for this segment,

Graph the hyperbola $\frac{x^{2}}{36} - \frac{y^{2}}{4} = 1$

Solution

As you can see, the hyperbola is already in the standard form.

$\frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1$

This means that we have a pair of curves opening from the left and right. The vertices are $(\pm 6, 0)$ and the foci are $(\pm 2 \sqrt{10}, 0)$ . The centre of the hyperbola is the origin, (0, 0). Here,

$a^{2} = 36 \Rightarrow a = 6 a n d b^{2} = 4 \Rightarrow b = 2$

The equation of the asymptotes are

$y = \pm \frac{b}{a} x = \pm \frac{2}{6} x = \pm \frac{1}{3} x$

Always sketch the asymptotes when graphing hyperbolas. That way, we can accurately draw the curves associated with the equation.

The graph of $\frac{x^{2}}{36} - \frac{y^{2}}{4} = 1$ is shown below.

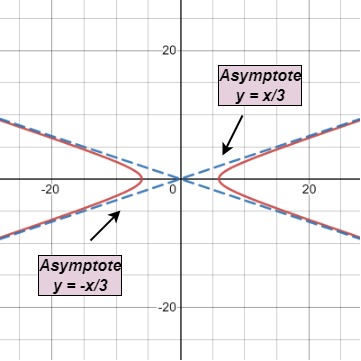
Graph an Equation Not in Standard Form

In this section, it may be helpful to recall the method of Completing Squares to solve such problems.

Graph the hyperbola below.

9 x^{2} - 25 y^{2} - 36 x - 50 y - 214 = 0

Solution

To solve this expression, we must attempt to rearrange this in the standard form of a hyperbola. We do this by completing the square as follows.

$9 x^{2} - 36 x - 25 y^{2} - 50 y = 214 9 (x^{2} - 4 x + A) - 25 (y^{2} + 2 y + B) = 214 + 9 (A) - 25 (B)$

We need to identify A and B. In doing so, we obtain

$9 (x^{2} - 4 x + 4) - 25 (y^{2} + 2 y + 1) = 214 + 9 (4) - 25 (1) \Rightarrow 9 {(x - 2)}^{2} - 25 {(y + 1)}^{2} = 225$

Dividing both sides by 225, we obtain the equation

$\Rightarrow \frac{{(x - 2)}^{2}}{25} - \frac{{(y + 1)}^{2}}{9} = 1$

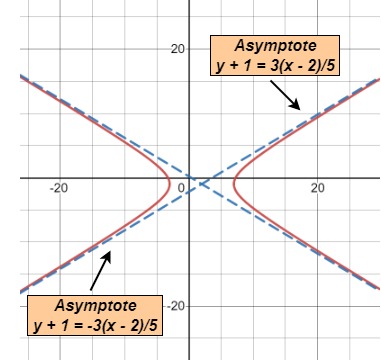
The centre is (2, –1). We also have the values $h = 2, k = - 1, a = 5, b = 3 a n d c = \sqrt{34}$ . Thus we obtain the following values for the vertices, foci and asymptotes.

The vertices

$(h \pm a, k) = (2 \pm 5, - 1) \Rightarrow (- 3, - 1) a n d (7, - 1)$

The foci

$(h \pm c, k) = (2 \pm \sqrt{34}, - 1) \Rightarrow (2 - \sqrt{34}, - 1) a n d (2 - \sqrt{34}, - 1)$

The asymptotes

$y = k \pm \frac{b}{a} (x - h) = - 1 \pm \frac{3}{5} (x - 2) \Rightarrow y + 1 = - \frac{3}{5} (x - 2) a n d y + 1 = \frac{3}{5} (x - 2)$

The graph of $\frac{{(x - 2)}^{2}}{25} - \frac{{(y + 1)}^{2}}{9} = 1$ is shown below.

The Eccentricity of a Hyperbola

The eccentricity of a conic section describes how closely related the curve is compared to a circle. The eccentricity is described by the variable e.

The eccentricity of a circle is zero, e = 0.

The eccentricity of a hyperbola is always more than 1, e > 1. The formula for finding the eccentricity of a hyperbola is given below.

Formula: Eccentricity of a Hyperbola

$e = \frac{\sqrt{a^{2} + b^{2}}}{a}$

The bigger the eccentricity, the less curved the conic section is.

Find the eccentricity of the hyperbola $\frac{x^{2}}{25} - \frac{y^{2}}{9} = 1$

Solution

Here, a² = 25 and b² = 9. Thus, the eccentricity of this hyperbola is given by

$a = \sqrt{25} = 5 \Rightarrow e = \frac{\sqrt{25 + 9}}{5} \Rightarrow e = \frac{\sqrt{34}}{5} \approx 1.17 (c o r r e c t t o t w o d e c i m a l p l a c e s)$

Hyperbolas - Key takeaways

To locate the vertices and foci given the equation of a hyperbola in standard form, we adopt the following steps:

Identify the location of the transverse axis

I f t h e e q u a t i o n i s o f t h e f o r m \frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1 \Rightarrow t r a n s v e r s e a x i s l i e s o n t h e x - a x i s a n d t h e v e r t i c e s a r e (\pm a, 0) a n d t h e f o c i a r e (\pm c, 0)

I f t h e e q u a t i o n i s o f t h e f o r m \frac{y^{2}}{a^{2}} - \frac{x^{2}}{b^{2}} = 1 \Rightarrow t r a n s v e r s e a x i s l i e s o n t h e y - a x i s a n d t h e v e r t i c e s a r e (0, \pm a) a n d t h e f o c i a r e (0, \pm c)

Solve for a using $a = \sqrt{a^{2}}$
Solve for c using $c = \sqrt{a^{2} + b^{2}}$

To express an equation in standard form given the vertices and foci for a hyperbola centred at (0,0), we use the method below:

Determine the location of the transverse axis

I f t h e v e r t i c e s a r e (\pm a, 0) a n d t h e f o c i a r e (\pm c, 0) \Rightarrow t r a n s v e r s e a x i s l i e s o n t h e x - a x i s a n d t h e e q u a t i o n i s o f t h e f o r m \frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1

I f t h e v e r t i c e s a r e (0, \pm a) a n d t h e f o c i a r e (0, \pm c) \Rightarrow t r a n s v e r s e a x i s l i e s o n t h e y - a x i s a n d t h e e q u a t i o n i s o f t h e f o r m \frac{y^{2}}{a^{2}} - \frac{x^{2}}{b^{2}} = 1

Solve for b² using b² = c² - a²
Substitute a² and b² into the standard form established in Step 1

To write an equation in standard form given the vertices and foci for a hyperbola centred at (h,k), we apply the technique below:

Decide whether the transverse axis is parallel to the x-axis or y-axis

I f t h e y - c o o r d i n a t e s o f t h e v e r t i c e s a n d f o c i a r e t h e s a m e \Rightarrow t r a n s v e r s e a x i s l i e s o n t h e x - a x i s a n d t h e e q u a t i o n i s o f t h e f o r m \frac{{(x - h)}^{2}}{a^{2}} - \frac{{(y - k)}^{2}}{b^{2}} = 1

I f t h e x - c o o r d i n a t e s o f t h e v e r t i c e s a n d f o c i a r e t h e s a m e \Rightarrow t r a n s v e r s e a x i s l i e s o n t h e y - a x i s a n d t h e e q u a t i o n i s o f t h e f o r m \frac{{(y - k)}^{2}}{a^{2}} - \frac{{(x - h)}^{2}}{b^{2}} = 1

Identify the centre of the hyperbola, (h, k) using the Midpoint Formula given coordinates of the vertices $(h, k) = (\frac{x_{1} + x_{2}}{2}, \frac{y_{1} + y_{2}}{2})$
Evaluate a² by solving the length of the transverse axis, 2a. This is given by the distance between the two vertices
Evaluate c² by using the coordinates of the given foci and the values of h and k determined in Step 2
Solve for b²
Substitute a², b², h and k into the standard form established in Step 1

Frequently Asked Questions about Hyperbolas

A hyperbola is the set of all points in a plane where the absolute value of the difference in distance between two fixed points, called the foci, is constant

The asymptotes for the general form of a hyperbola is given by y = ± bx/a

Find the centre of the hyperbola
Locate the vertices and construct the corresponding vertical lines
Determine the co-vertices and construct the corresponding horizontal lines
This creates a rectangle
Draw two diagonal lines for this rectangle. These are the asymptotes of the hyperbola
Sketch the curves

Decide whether the transverse axis is parallel to the x-axis or y-axis
Identify the centre of the hyperbola, (h, k) using the Midpoint Formula given coordinates of the vertices
Evaluate a² by solving the length of the transverse axis, 2a. This is given by the distance between the two vertices
Evaluate c² by using the coordinates of the given foci and the values of h and k determined in Step 2
Solve for b²
Substitute a², b², h and k into the standard form established in Step 1

A hyperbola is a type of conic section made up of two parabola-like shaped curves. These pairs of curves can either open up and down or left and right. Additionally, each curve contains a vertex. However, it is crucial to note that these curves are not exactly parabolas (they only resemble them!)

Flashcards in Hyperbolas15 Start learning

What is the general form of a hyperbola centred at the origin?

\[ \frac{x^2}{a^2} - \frac{y^2}{b^2} = 1 \] or \[\frac{y^2}{a^2} - \frac{x^2}{b^2} = 1 \].

What is the general form of a hyperbola centered at $(h, k)$?

\[ \frac{(x-h)^2}{a^2} - \frac{(y-k)^2}{b^2} = 1 \] or \[\frac{(y-k)^2}{a^2} - \frac{(x-h)^2}{b^2} = 1 \].

How do you describe a hyperbola?

A hyperbola is the set of all points in a plane where the absolute value of the difference in distance between the foci is constant.

How many axes of symmetry does a hyperbola have? What are their names?

A hyperbola has two axes of symmetry: the transverse axis and the conjugate axis.

What is the transverse axis?

The transverse axis is a line that passes through the centre of the hyperbola. The foci lie on this line and have vertices as their endpoints.

What is the conjugate axis?

The conjugate axis is a line perpendicular to the transverse axis and has co-vertices as its endpoints.

Learn with 15 Hyperbolas flashcards in the free StudySmarter app

Already have an account? Log in

More about Hyperbolas

Save Article

How would you like to learn this content?

Creating flashcards

Studying with content from your peer

Taking a short quiz

How would you like to learn this content?

Creating flashcards

Studying with content from your peer

Taking a short quiz

Free math cheat sheet!

Everything you need to know on . A perfect summary so you can easily remember everything.

Email Address*

Find Study Materials for

Create Study Materials

Hyperbolas

Geometrical Components of a Hyperbola

Equations of Hyperbolas

Properties of Hyperbolas